Do it yourself European Unemployment Insurance
To illustrate our recent Policy Brief, this blog post proposes to give the reader the opportunity to take the main decisions on the design of a Europe
In their Policy Brief prepared for the September 13 informal meeting of EU finance ministers (ECOFIN), Claeys, Darvas and Wolff (2014) discuss the benefits, drawbacks and possible designs for a potential European Unemployment Insurance (EUI) scheme. They end their discussion by formulating 10 key decisions that policy makers would have to take before implementing such a scheme.
To illustrate this Policy Brief, this blog post proposes to give the reader the opportunity to make the main decisions on the design of an EUI scheme. Given that one of the main purposes of an EUI scheme would be to act as a stabilization mechanism by providing resources to the countries with increasing unemployment rates, these simulations allow you to see what would have been the potential outcomes of such a scheme if it had existed since 2000 depending on the various design parameters.
The decisions to take concerning the parameters are the following:
- At what level should the scheme be implemented: at the European Union (EU) or at Euro Area (EA) level?
- Should it be an ‘all-time” basic unemployment insurance (that would replace partly or fully national schemes) or a ‘catastrophic’ one (which would only be activated when there is a significant increase in the unemployment rate in a country)? In the case of a catastrophic insurance, what should be the trigger to activate it?
- Should the scheme try to be neutral over the cycle for each country (thanks to differentiated contribution rates across countries) or should it allow potential persistent transfers (with a single rate across the EU/EA)?
- What should be the replacement rate (i.e the share of the previous wage received as unemployment benefit)?
In order to simplify the choices for the non-expert reader, another possibility offered by our simulation tool is to run simulations by choosing directly the objectives you want the scheme to achieve rather than the exact parameters:
- Again, you will have to choose at what level the scheme should be implemented: European Union or Euro Area?
- What should be the degree of stabilization achieved by the scheme? Do you want it to deal with all shocks, just large shocks, or only very large shocks?
- What should be the degree of solidarity achieved by the scheme? Do you want a scheme that minimizes lasting transfers across countries or not?
For the interested readers, the methodology used for the simulations can be found at the end of this post.
[chart-content]
How to read the table, map and graph?
The table resulting from your design choices displays net payments as a percentage of GDP that would have been received by the countries from an EUI scheme since 2000. Positive numbers, in green, represent net payments to the countries, whereas negative numbers, in red, represent net contributions to the EUI scheme. The last 4 rows of the table indicate annual and cumulative cash positions of the scheme for each year (in % of GDP and in € billions). Positive numbers, in green, represent positive cash positions of the scheme that could be saved for the future, whereas negative numbers, in red, represent negative cash positions that would have to be financed by borrowing in the capital markets.
Try out some combinations and either choose which countries you want to be displayed on the chart or move the slider of the map through the years to visualize more clearly which member states receive what in each year. Remember green is equal to receiving payments from the scheme, while red means that the country is making net payments into the scheme.
The most interesting combinations
To help you get a flavour for what is possible to achieve with an EUI scheme depending on its design, let’s discuss three combinations that we think are interesting:
The basic scheme
In this variant, the insurance scheme provides benefits to all unemployed of the Euro area and is financed with a single contribution rate paid by all workers of the Eurozone, regardless of the previous use of the scheme and the current economic situation of their countries. The replacement of previous wages is limited to 50% (but countries with higher replacement rate can top that up with their own national schemes). Basically this variant of the scheme would replace partly the national unemployment schemes and net payments received by country would be directly proportionate to the number of unemployed.
As the result, this scheme provides permanent transfers from countries with low unemployment rate to those with high ones: Austria, the Netherlands and Luxembourg would have made net payments into the scheme for the whole of 2000-2013 while Finland, Greece and Spain would have been net receivers throughout the same period. On top of that the stabilization function would be limited: for instance, in net terms, Spain would have received on average less than 1% of its GDP per year from 2009 to 2013 to pay the unemployment benefits of its newly unemployed citizens. The main goal of such a scheme would therefore be to establish some solidarity link between workers of the Eurozone.
The catastrophic scheme
This variant of the scheme sets a single contribution rate, just like the previous arrangement, and also applies only to Euro Area countries. However, this time, the replacement rate is a generous 80% (the best possible in the simulation) but this generosity comes with conditions. The catastrophic insurance scheme is only activated when unemployment rises in a year by more than 0.5 percentage points above its previous 5-year average.
Such a scheme would be much more countercyclical. Faced with a high increase of its unemployment rate since the burst of its housing bubble, Spain would have received on average 2.2% of its GDP per year from 2008 to 2013, because of the huge increase of its unemployment rate due to the bursting of its housing bubble. However, another interesting feature of this variant of the scheme is that many countries would have been net receivers since 2000 when they experienced bad times: Austria in 2004-05, Belgium in 2003-04, Cyprus and Greece since 2009, Germany and the Netherlands in 2003-05, etc. This variant of the scheme would therefore fulfil the main goals assigned to a European Unemployment Insurance scheme fairly well: provide a stabilization mechanism against asymmetric shocks and enhance European solidarity without creating transfers between countries that are too large or too persistent. Therefore, it constitutes one of our favourite EUI combinations available in the simulations. Nevertheless, one potential drawback of such a scheme is that it would have accumulated a non-negligible debt during the crisis that started in 2008-09, equivalent to 2.3% of the Eurozone GDP at the end of 2013, because some borrowing (the equivalent on average of 0.37% of the EA GDP per year) would have been necessary since 2009 to finance the scheme.
The borrowing capacity scheme for catastrophic times
This variant makes the scheme lighter than the previous arrangement by allowing the contribution rates to be different in each country and also by increasing the activation trigger of the scheme to 1.5 percentage points. Countries that resort to the scheme in bad times will end up paying higher contributions in better times to reimburse the debt of the scheme, whereas countries not using the scheme will not have to contribute to its financing. Given to the lack of direct transfers, this variant looks less like an risk-sharing mechanism and more like a pan-Euro area/EU borrowing facility that countries can use in case of a catastrophic increase of their unemployment rates. The idea would be that the scheme would be guaranteed by all members of the scheme or by the EU itself to benefit from a good rating and lower interest rates.
This variant of the scheme would rarely be used: only nine countries would have resorted to the scheme since 2000 and only two (Luxembourg and Portugal) would have before 2008. This version of the scheme would preclude permanent transfers across countries by construction and would be activated only in the worst times, so it would be quite countercyclical (although less than the previous version as the contributions paid in the troubled countries would increase more and more quickly, given that the financing would not be mutualised). This combination could be a good option to provide a stabilization mechanism to the monetary union if countries absolutely want to avoid transfers across countries.
Methodology and assumptions used in the simulation (wonkish!)
The approach used in this simple simulation exercise is quite similar to the one used by Lellouch and Sode (2014) and Epaulard (2014). For the sake of simplicity, we make some assumptions that suffer from several limitations. The main limitation being that our simulation takes unemployment and GDP as exogenous variables and uses historical values. Thus, it does not take into account the positive feedback (resulting from potentially high multiplier effects, especially during recession) that could have ensued from the existence of such a scheme in terms of higher GDP and lower unemployment. This is therefore different from an econometric simulation, in which the effects of the payments by the EUI scheme would be generated in a macro model, which would include indirect feedback effects. However, we still think that our simulations are useful to give the reader an idea about the differences in the payment flow magnitudes of the various potential schemes.
For the interested reader, the following sections explain precisely the calculations behind the simulations:
Expenditures of the EUI scheme:
For country i and year t, when the scheme is activated (i.e. every year in the ‘all-time’ variant of the, and only in the years during which unemployment increase significantly in the ‘catastrophic’ variant), its expenditures are calculated as follows:

The components of expenditures are defined as follows:
- Average Wage before being unemployed: Data on former wages of the unemployed is not available directly, which forces us to make some assumptions to approximate it. Given that lower qualification and lower wage workers are often more likely to be unemployed, a possible solution to proxy the average wage of the currently unemployed before they lost their job is simply to multiply the average wage of those currently in work by a constant k, with 0< k<1. Following Dullien (2013), we assume in our simulations a constant value of k equal to 0.8, meaning that the average former wage of the unemployed would be equal to 80% of the average wage in the economy. Therefore we have:
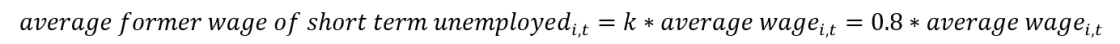
- Coverage Ratio: The coverage ratio is the share (in %) of the total number of short-term unemployed that are actually receiving benefits. The coverage ratio used in the simulation can be constructed in one of two ways – with historical data, or in a discretionary way.Using historical data would allow the simulation to take into account the heterogeneity of labor market institutions across countries. However, in order to consider the commonality of the eligibility criteria that would have resulted from the implementation of a European Unemployment Insurance scheme in 2000, it would not make sense to use directly historical values. A solution would be to estimate virtual coverage ratios for each country that would lie halfway between the historical rates and the European Union/Euro area average. A major problem with that solution is that a lot of data on the coverage ratio is missing. For some countries the entire time series is not available, or for other countries the series are very short and/or values are missing. This would imply that we would have to make some complex assumptions about what the coverage ratios would have been in these countries.Given these caveats, the other (‘discretionary’) solution is to set the coverage ratio at the same level for all countries at all times. In fact, as visible in chart 7 of Lellouch and Sode (2014), there exists a clear relationship between the strictness of eligibility criteria and the coverage ratios. Thus, with a common set of eligibility rules at the EU/EA level, it is probable that coverage ratios would converge across countries.For the sake of simplicity, we assume in our simulations that the adjustment is immediate when the scheme is implemented in 2000 and we choose a constant value equal to 40% for the coverage ratio of the EUI scheme, close to the current EU/EA average. In a way, this would mean that the eligibility criteria chosen for the European scheme would be comparable to the current average criteria of the EU/EA. Therefore we now have:
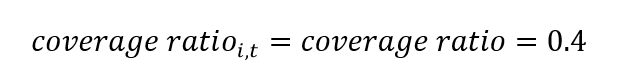
- Nevertheless, this would not imply necessarily that a race to the bottom for the above-average countries would be taking place. More generous national systems (that tend to have higher coverage ratios) would be able top up the European scheme: workers complying with looser national rules would remain covered by their country (implying that total coverage ratio – i.e. European plus national – could be higher than the European one).
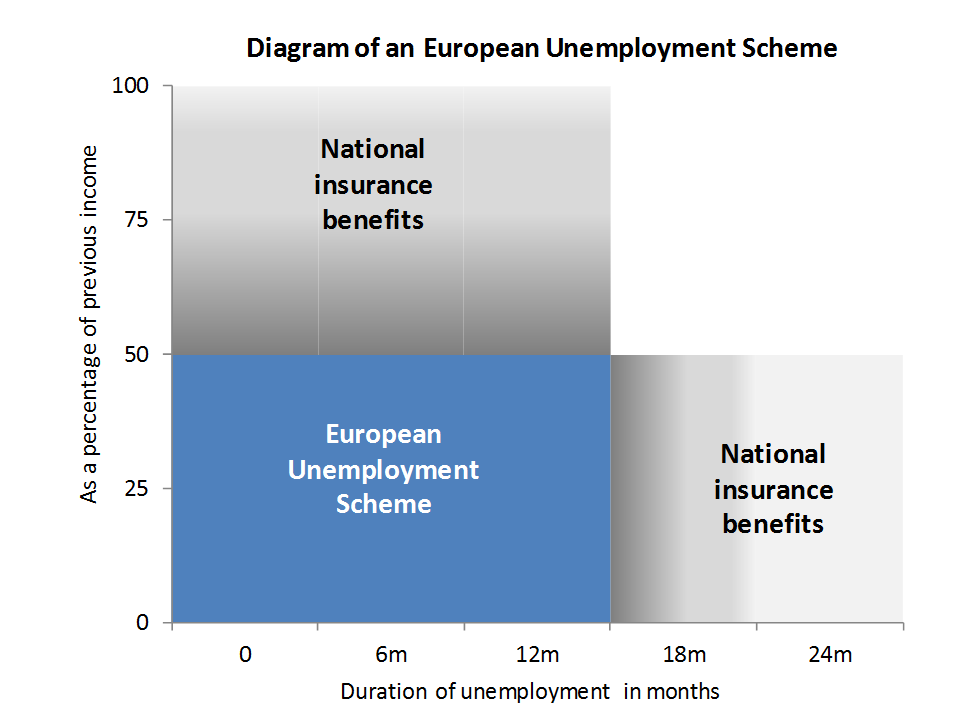
- Replacement rate: The replacement rate is the share (in %) of the former wage received as unemployment benefit. This is one of the main policy choices in our simulation and its value can be chosen between 50% and 80% to make the scheme more or less generous (this range of replacement rates is close to the one currently prevailing in Europe, see Table 1 in Claeys et al., 2014 for more details). Depending on the chosen value, countries with currently higher replacement rates would be able to top up the European scheme if they wanted to (see diagram below to visualize how the European and the national schemes would be organized). However, note that for 8 countries of the EU, a 50% rate would already be higher than what they currently pay to their unemployed citizens.
Source: Bruegel based on Dullien (2012)
Resources of the EUI scheme:
For country i and year t, resources of the EUI scheme are defined as follows:
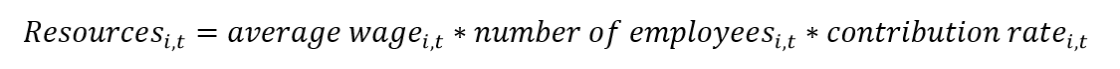
or more simply:
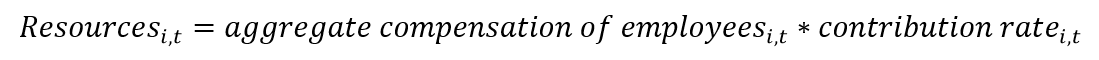
The main issue now is to decide how to set the contribution rates. Of course, the best way to set those rates would be to evaluate the probabilities of each countries to be affected by all possible shocks leading to a raise in unemployment in order to have an ex ante neutral scheme. However, this is in practice impossible to do. Even though less satisfying from a theoretical point of view, a more realistic solution is therefore to set contribution rates from an ex post perspective in a way that would possible in real-time.
For ‘all-time’ insurance, the scheme is active every year. In the case in which we want to minimize persistent transfers, contribution rates are differentiated across countries and determined in a way that would have balanced the fund at the country level over the past five years. This contribution rates would be updated each year.
In the case of differentiated rates, the problem in year t is therefore the following:
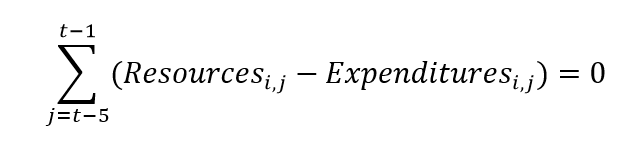
and therefore the contribution rates are defined as:
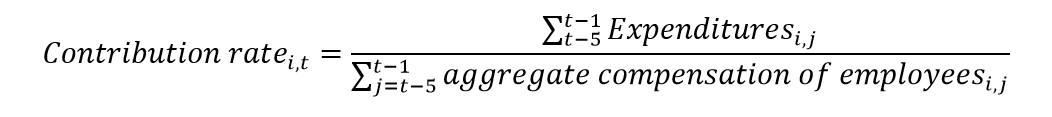
To increase the solidarity of the scheme and its stabilization properties a unique rate for all countries can be considered. In order to avoid a persistent negative cash position of the scheme after a crisis, this single contribution rate could be set up such that it would have balanced the fund at the area level over the past five years, and would also be updated each year.
In the case of a single rate, the problem becomes the following:
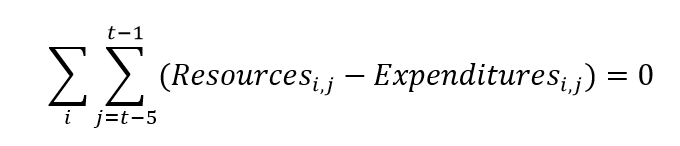
And the contribution rate is therefore defined as:
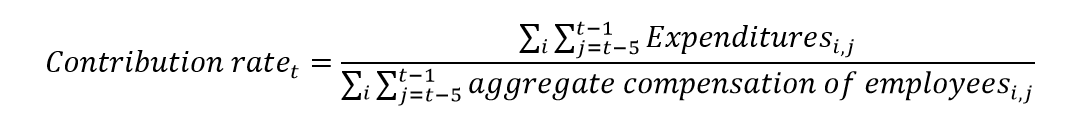
If ‘catastrophic’ insurance is chosen, the scheme is activated only when short-term unemployment rises by more than 0.5, 1 or 1.5 percentage points above its average over the past five years (these values are the therefore potential triggers of the scheme).
As before, contribution rates can also be differentiated, so that each country’s contribution take into account its prior use of the scheme (with the same calculation method as before with a rate that would have balanced the fund at the country level over the past five years). In that case, countries that persistently resort to the scheme in bad times will end up paying more in better times, whereas a country not using the scheme will not have to contribute to its financing, thanks to the borrowing capacity of the scheme. Note there can be gross flows can go in both directions at the same time. For example, if a country has resorted to the scheme, its contribution rate will increase, gradually increasing its gross payment, but if its unemployment rate continues to increase quickly, this would activate further inflows from the scheme simultaneously.
A version with a single rate paid by all countries (regardless of the individual use of the scheme) can also be considered with a ‘catastrophic’ scheme. Again, the contribution rate would be set every year at the level that would have balanced the fund at the area level over the past five years in order to avoid a lasting negative cumulative cash position of the scheme. The calculation of the single contribution rate would again be the same as the one used in the ‘all-time’ variant of the scheme.



